Ideal Gaussian noise theoretically has an unlimited range, meaning its amplitude can extend to infinity, though the probability of extremely large values is infinitesimally small. However, in practice, generating true Gaussian-distributed noise with electronic noise signal generators is nearly impossible due to the inherent limitations in output range of all devices. Therefore, it is necessary to implement a noise generator that closely approximates Gaussian-distributed noise.

Generating uniformly distributed random numbers is straightforward and easily implemented in FPGA systems. For instance, the linear-feedback shift register (LFSR) is a widely used algorithm for generating pseudo-random numbers with a uniform distribution. However, to achieve Gaussian-distributed noise, it is necessary to transform the uniform distribution into a Gaussian distribution.

The addition of two uniform distributions results in the convolution of those distributions. Adding random variables in the time domain is equivalent to performing convolution on the probability density functions of the random variables. For more details, please refer to this link.

The convolution of two uniform distributions results in a triangular distribution:

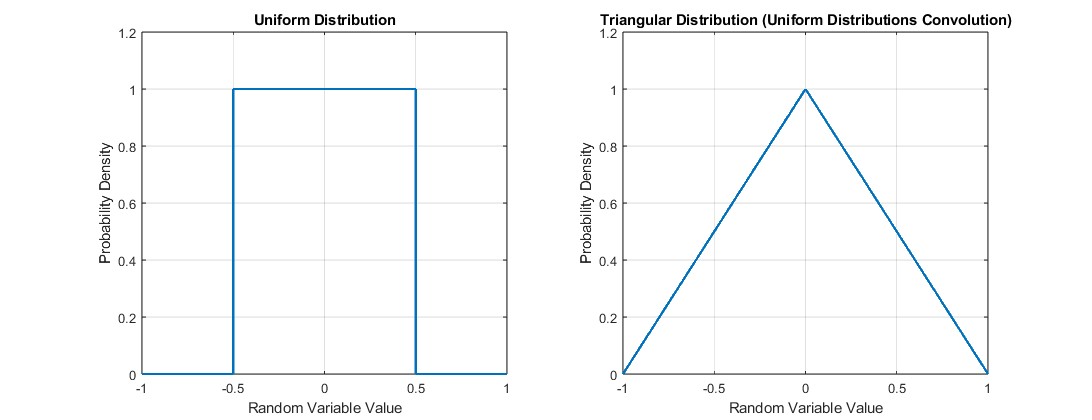
And the convolution of two triangular distributions results in a Gaussian-like distribution:

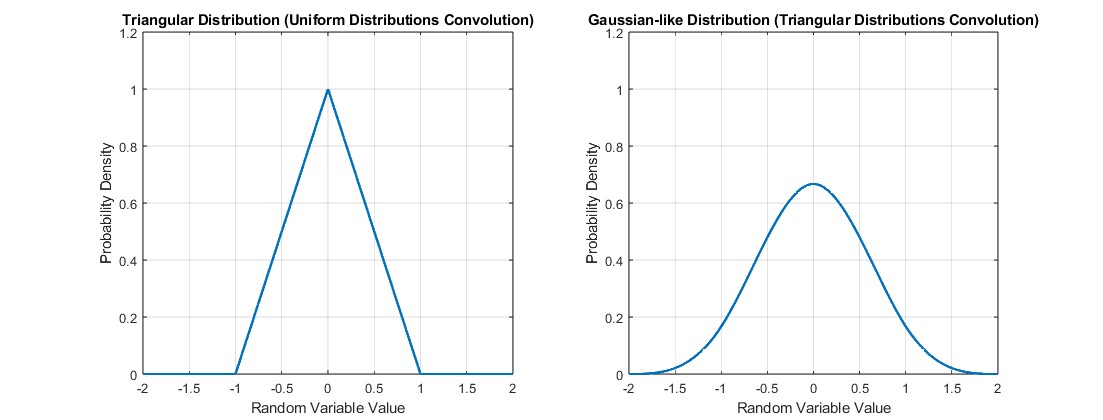
A true Gaussian distribution with σ = sqrt(1/48) has been generated to compare it with the rescaled Gaussian-like distribution. It can be seen that the Gaussian-like distribution reasonably approximates a true Gaussian distribution.

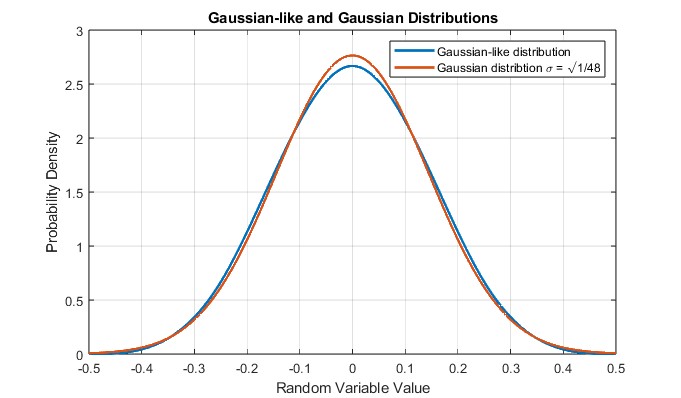
The variance of a uniform distribution with a range of $[-0.5,0.5]$[−0.5,  0.5] is 1/12. The addition of four independent uniformly distributed random variables will result in a new random variable with a variance of $\frac{4}{12}$4/12. However, the range of this new variable expands from $[-0.5,0.5]$[−0.5,  0.5] to $[-2,2]$[−2,  2]. To maintain a unity range, this variable needs to be scaled down by a factor of 4. Consequently, the variance of the rescaled random variable is 1/48​. Therefore, the standard deviation of the rescaled random variable is sqrt(1/48)​.

For example, when the Moku Waveform Generator outputs a 1 Vpp noise waveform, the minimum and maximum values of the signal are -0.5 V and 0.5 V, respectively. The standard deviation of the 1 Vpp noise signal is approximately 144.338 mV, which is sqrt(1/48).